Classical Mechanics
Course Notes (Marko Horbatsch)
Text: Jens
M. Knudsen, Poul G. Hjorth: Elements of Newtonian Mechanics, 3rd
edition, Springer-Verlag 2000
1
Newtonian Mechanics in one dimension
1.1 Introduction Free Fall Definite Integration
1.2 Harmonic
Oscillator Total Energy Visualize Motion
1.3 Damped Harmonic
Motion Weak and Strong Damping Critical Damping Energy in the Damped HO
1.4 Driven Harmonic
Oscillator Solution Discussion
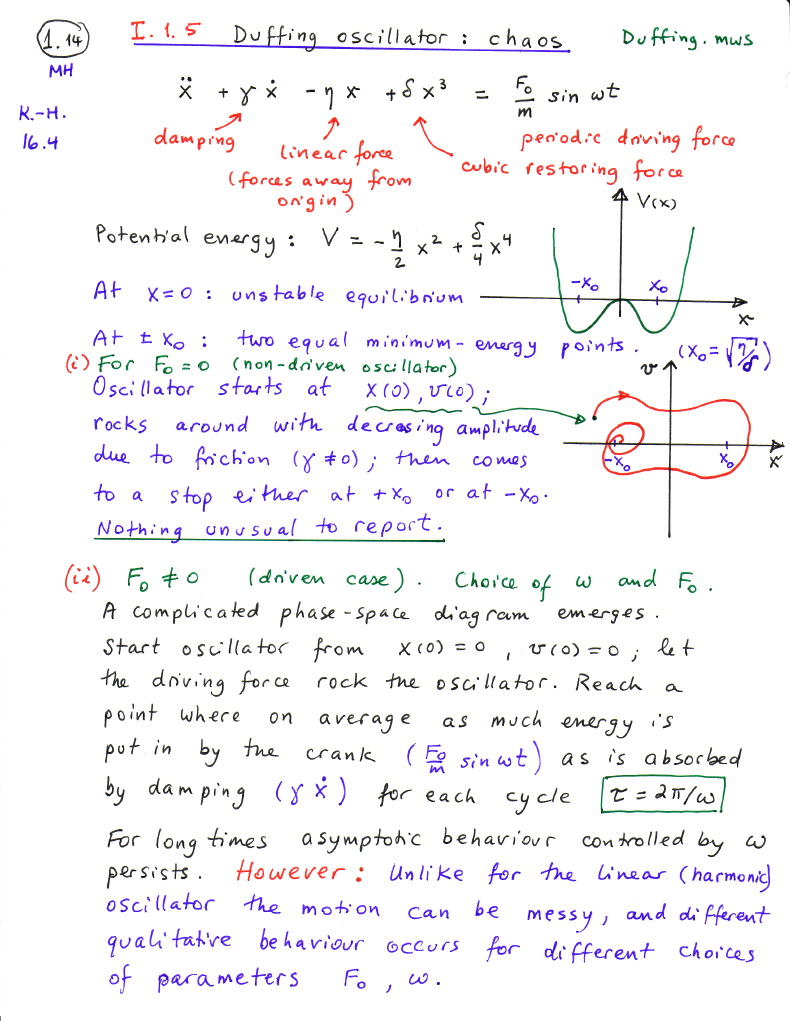
1.5 Chaos in the
Duffing Oscillator Poincare Section
1.6 Work and Energy
in1D Motion
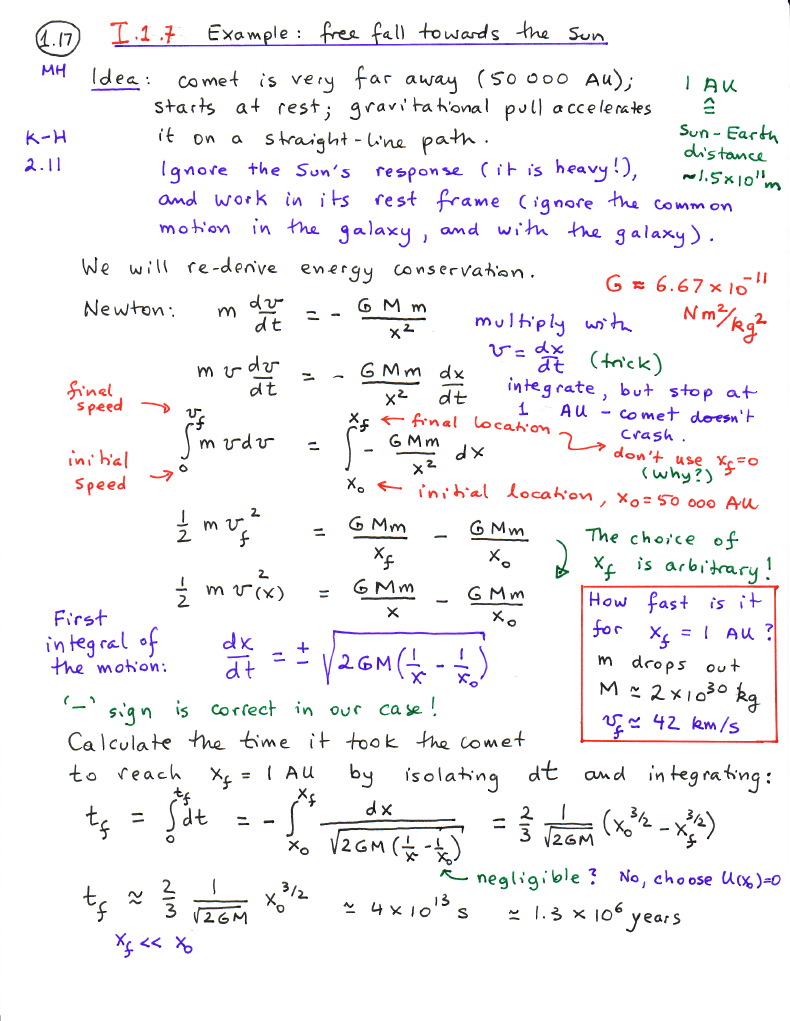
1.7 Example: Free
Fall towards the Sun
1.8 Conservation of
Linear Momentum
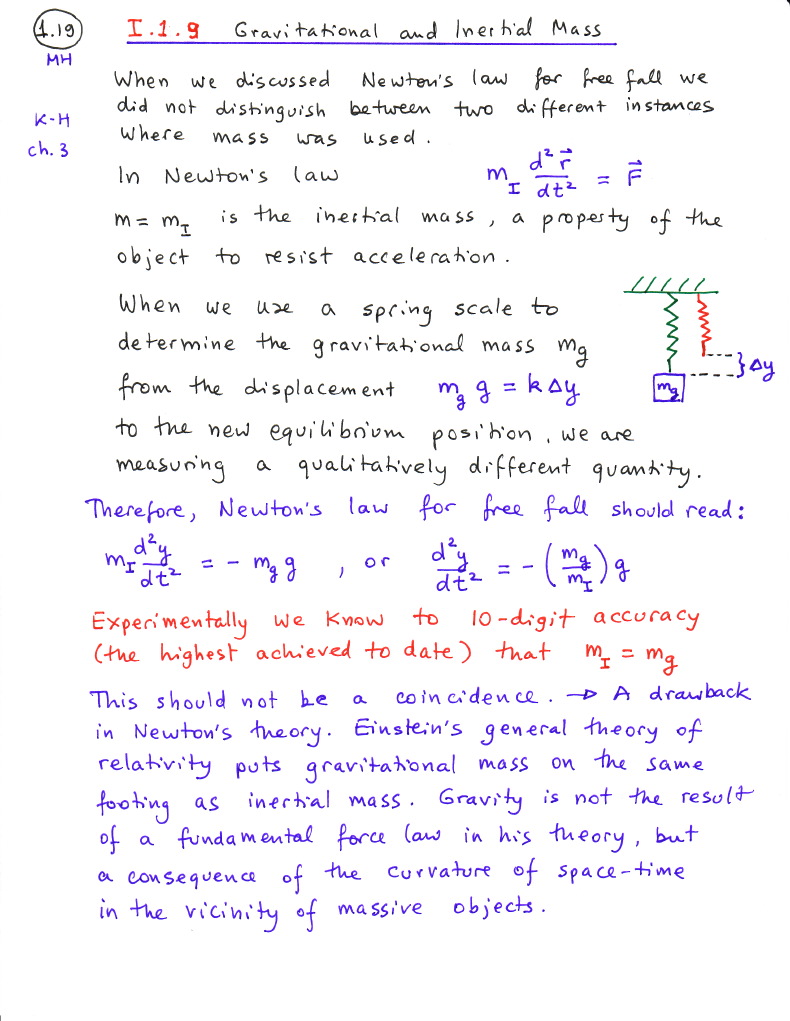
1.9 Gravitational and
Inertial Mass
1
Mechanics in three dimensions
2.1 Charged Particle in a Magnetic Field Solution
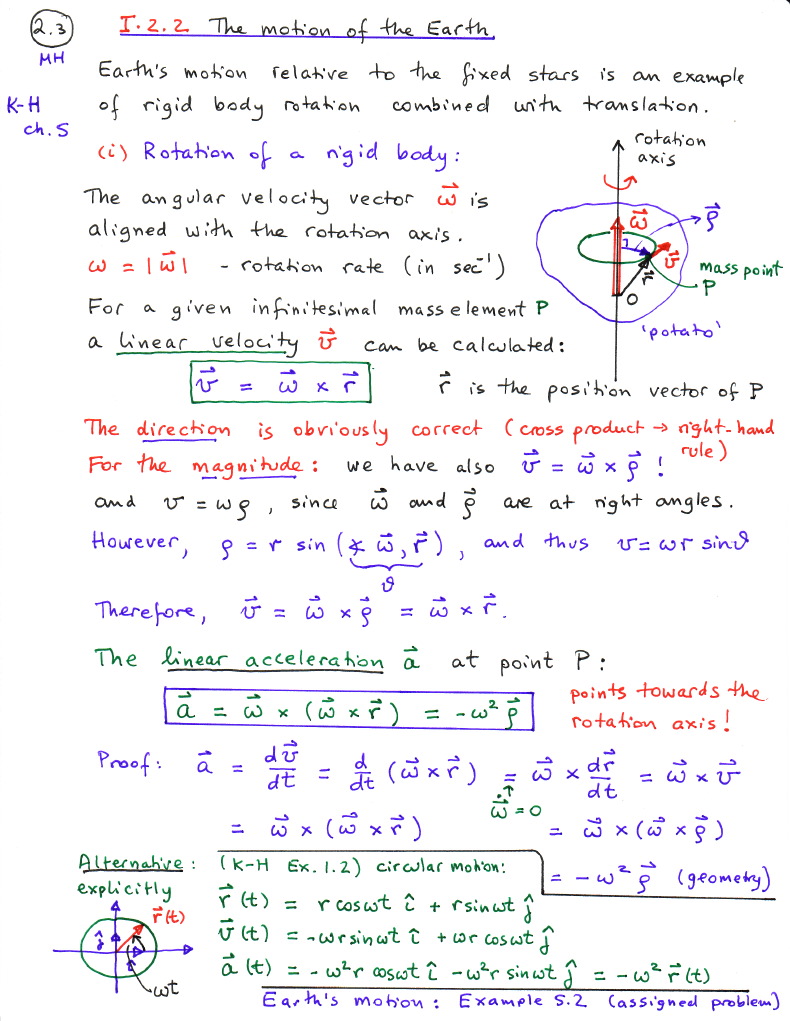
2.2 Earth’s Motion Cross Product Test1Problem1
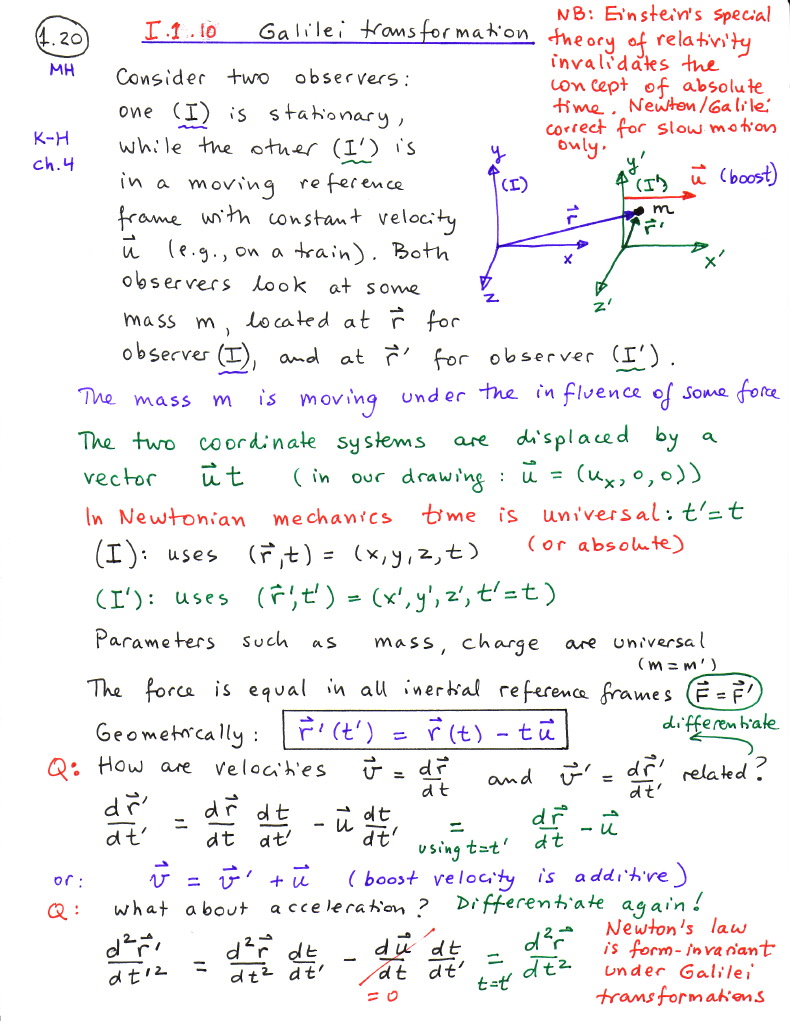
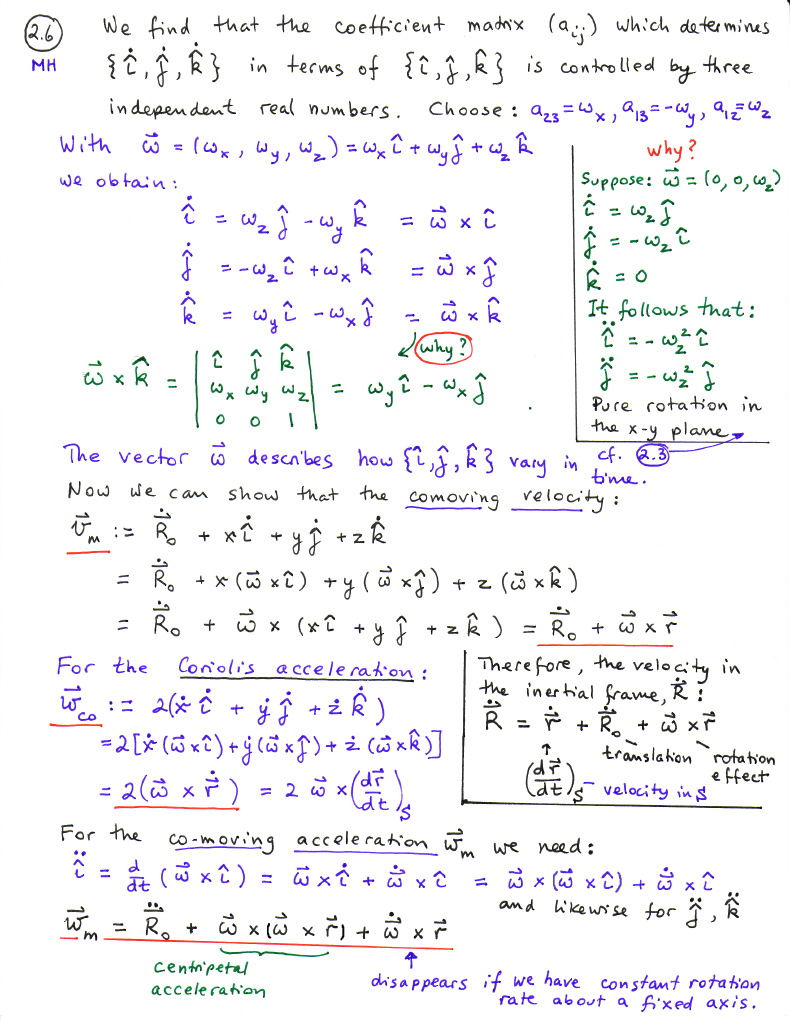
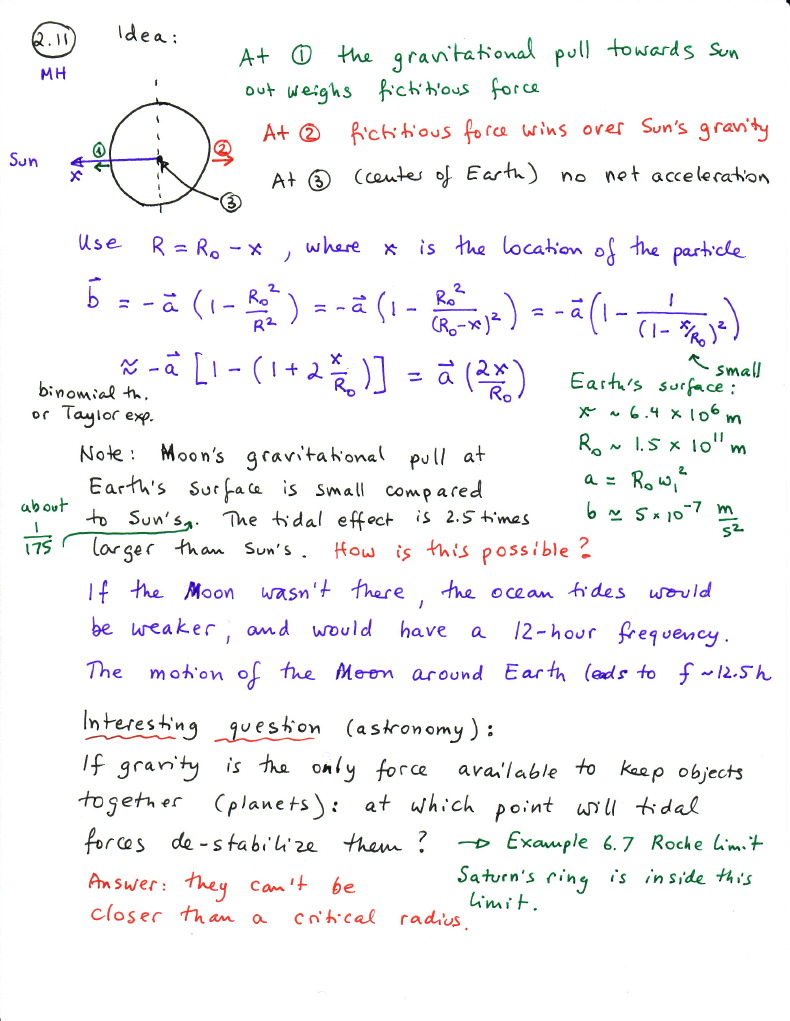
2.3 Accelerated Reference Frames Transformation Transformation(…) Transformation Results
2.4 Einstein’s Equivalence Principle
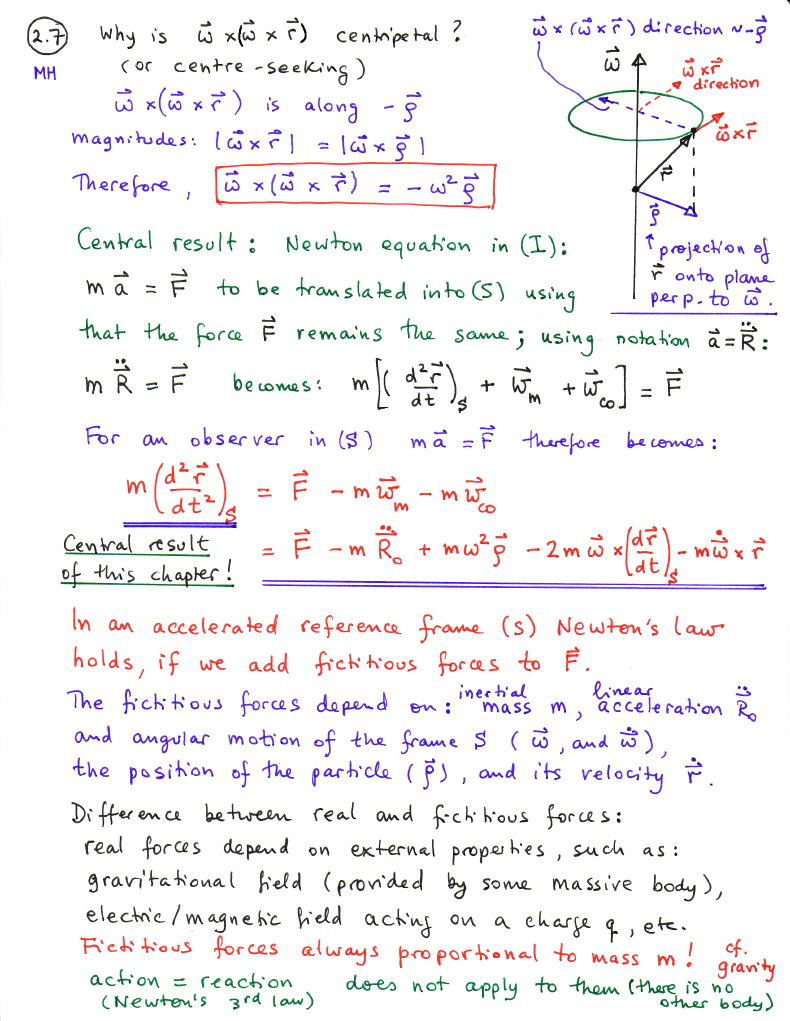
2.7 Coriolis Force
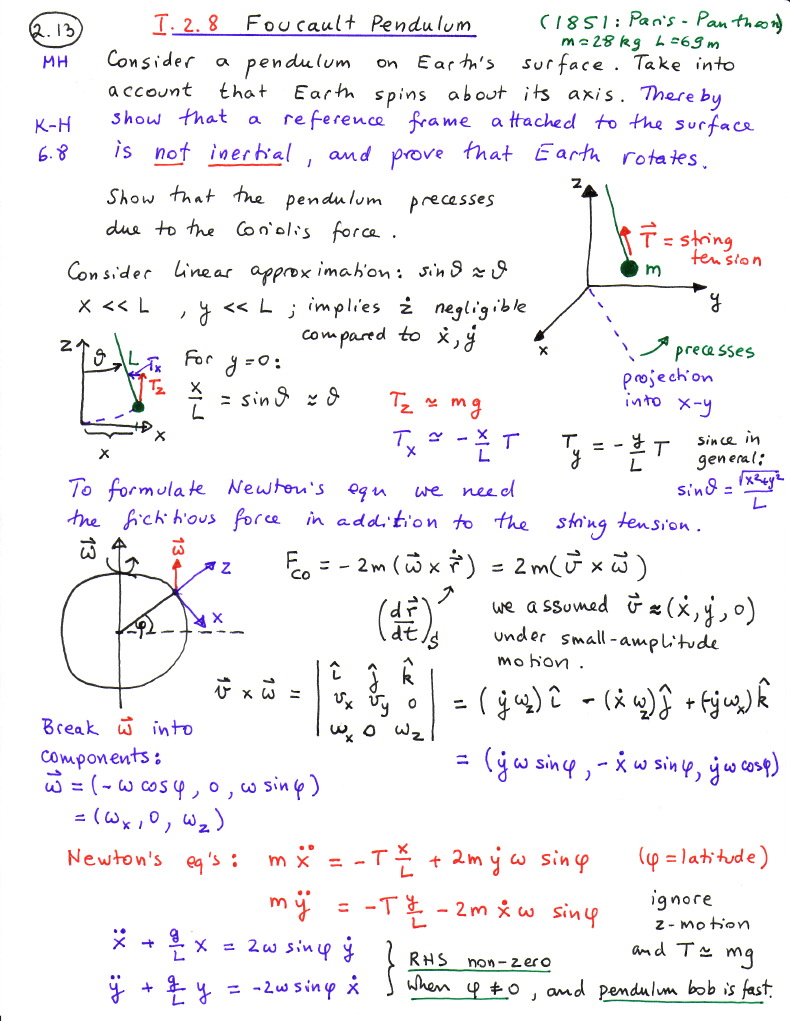
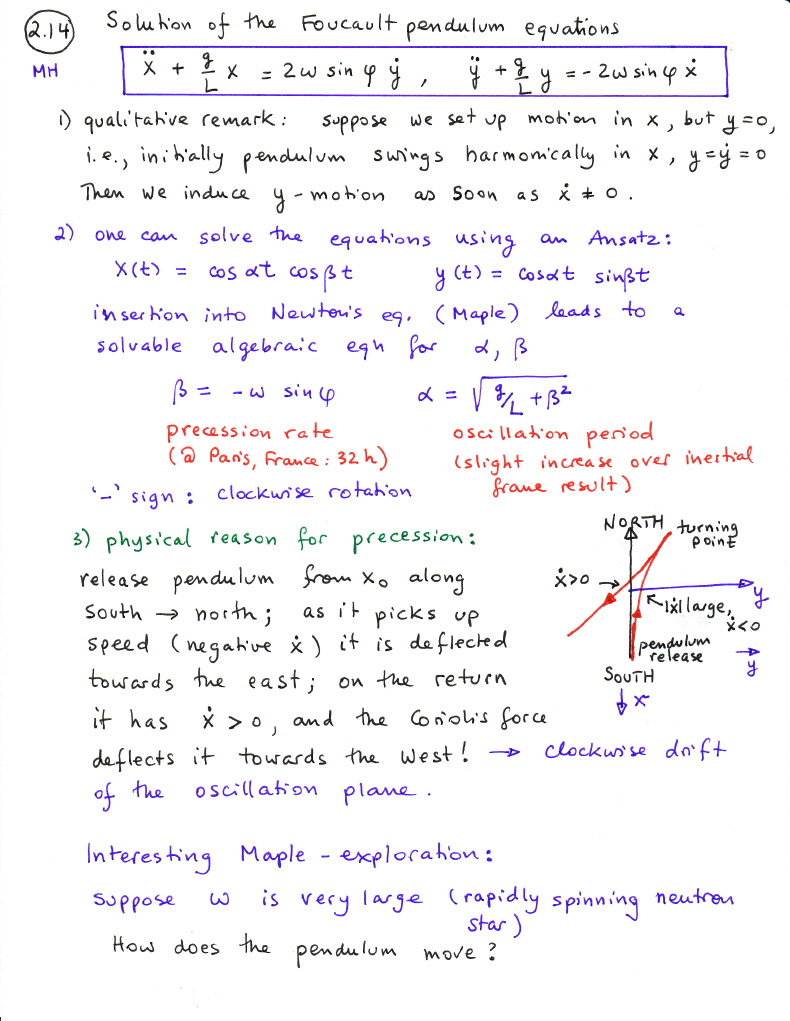
2.8 Foucault Pendulum Solution
2
Advanced Concepts
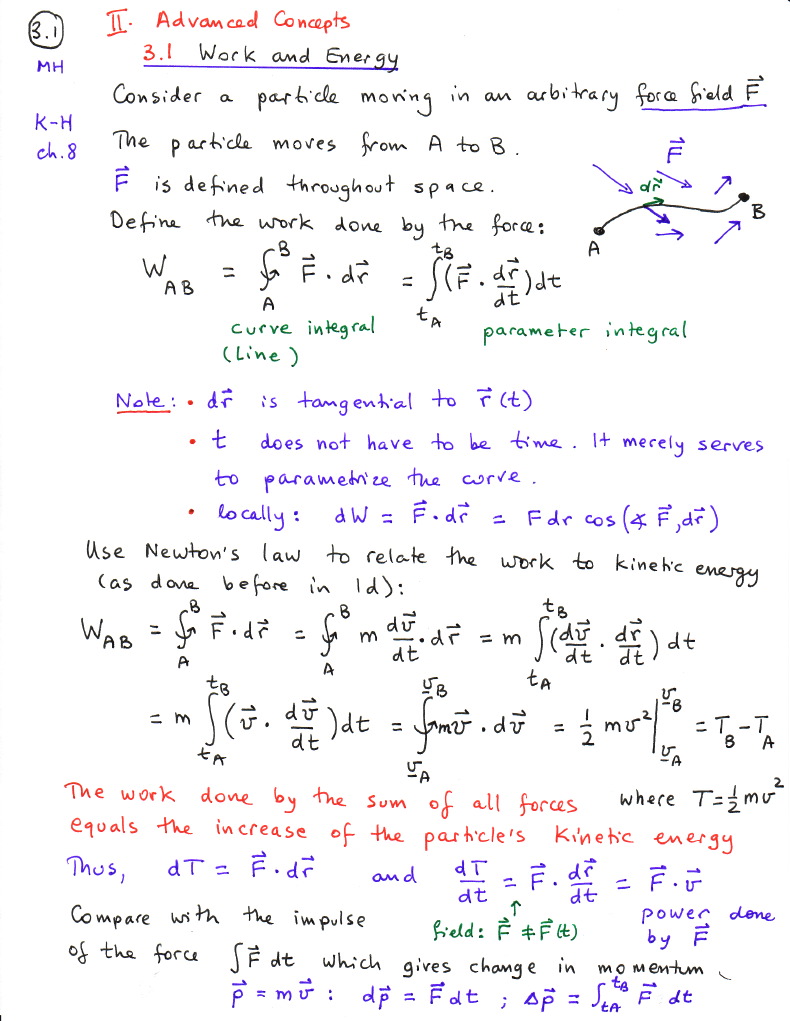
3.1 Work and Energy
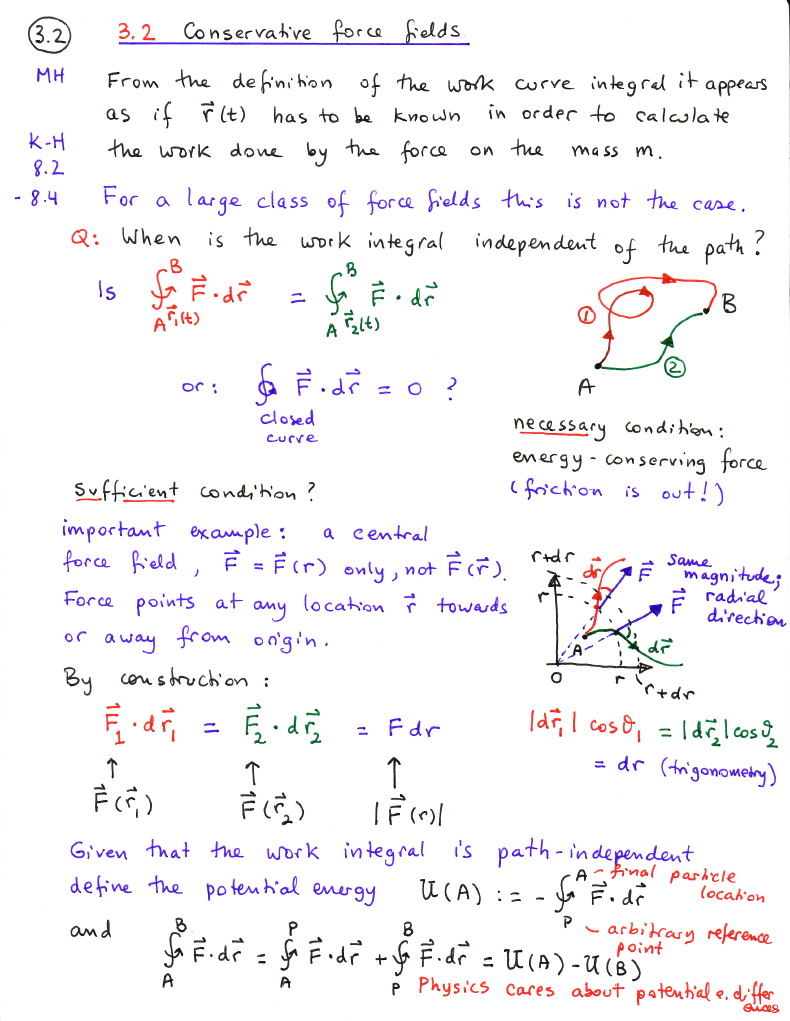
3.2 Conservative Force Fields curl F = 0 Stokes’ Theorem
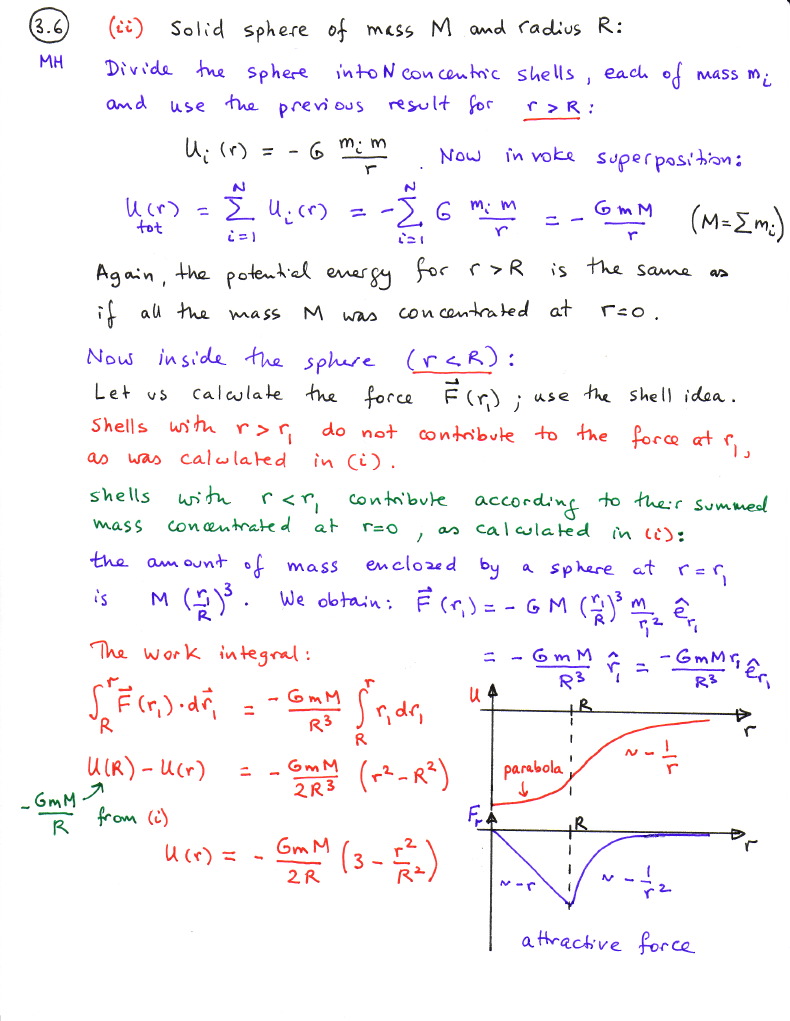
3.3 Gravitational Potential Energy Calculation Solid Sphere Self-Energy of the Sun (Pr 8.9)
Summary: Problem-Solving: 6.1,8.2,8.3, 8.8
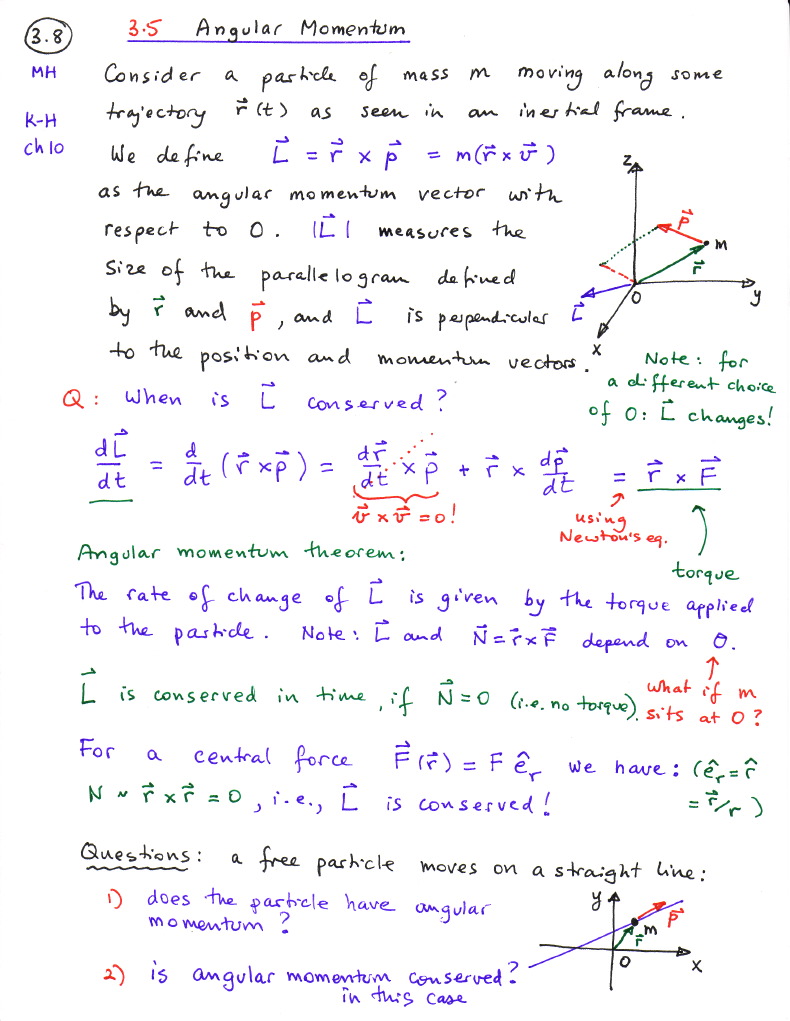
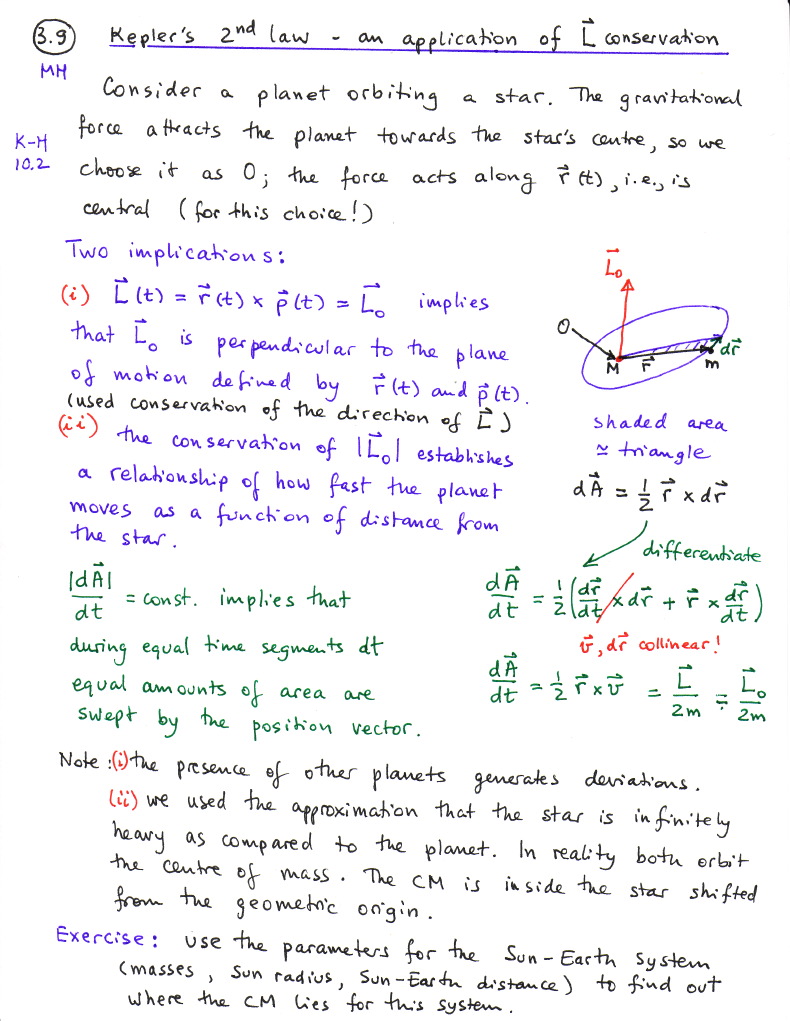
3.5 Angular Momentum Kepler’s 2nd Law
3.6 Effective Potential in the Kepler Problem
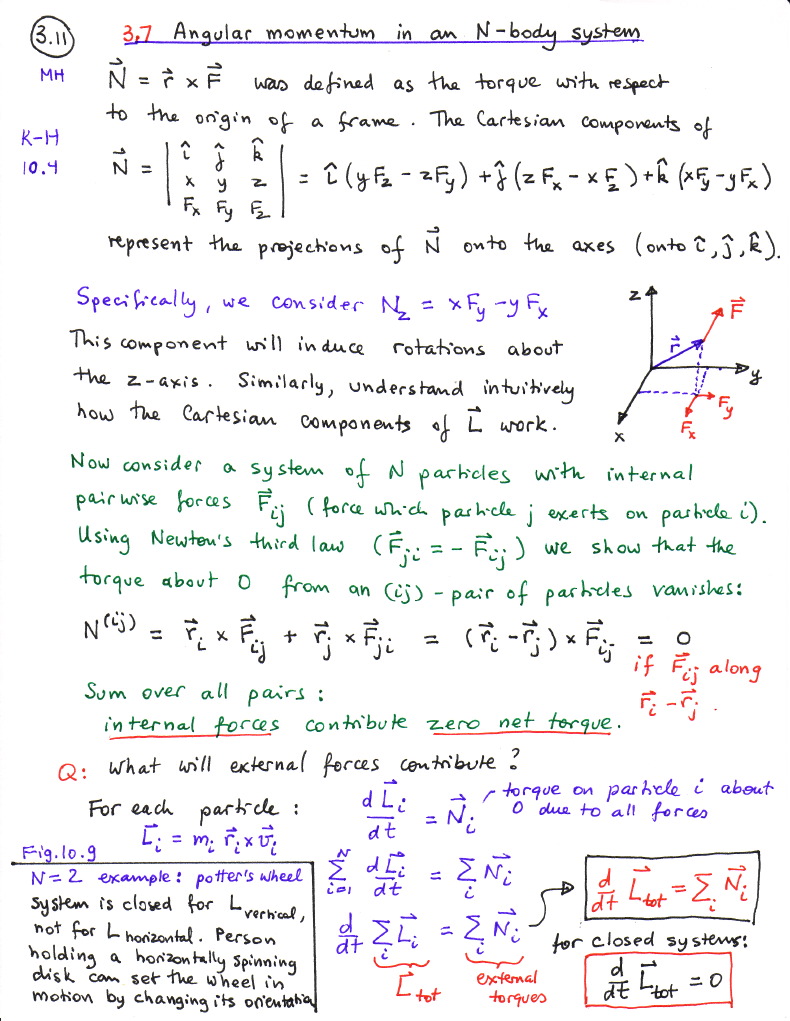
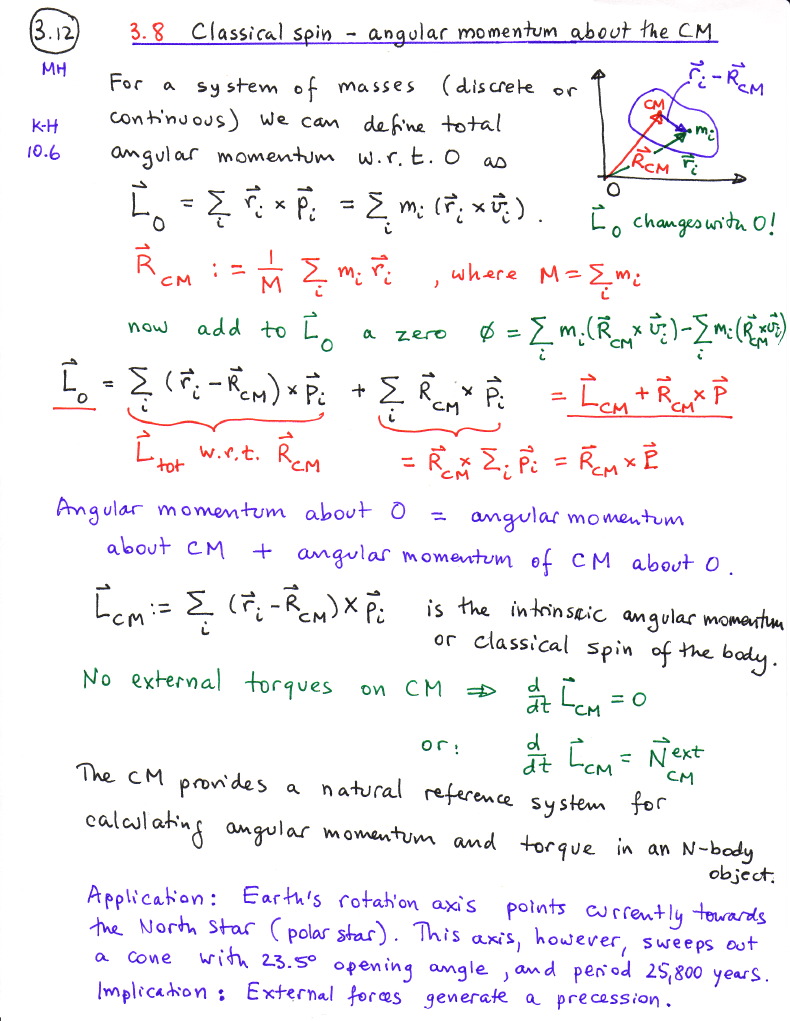
3.7 Angular Momentum in a Many-Body System
3.8 Classical Spin of a Rigid Body
3
Rotational Rigid-Body Motion
4.1 Rigid-body rotation basics Rotational kinetic energy
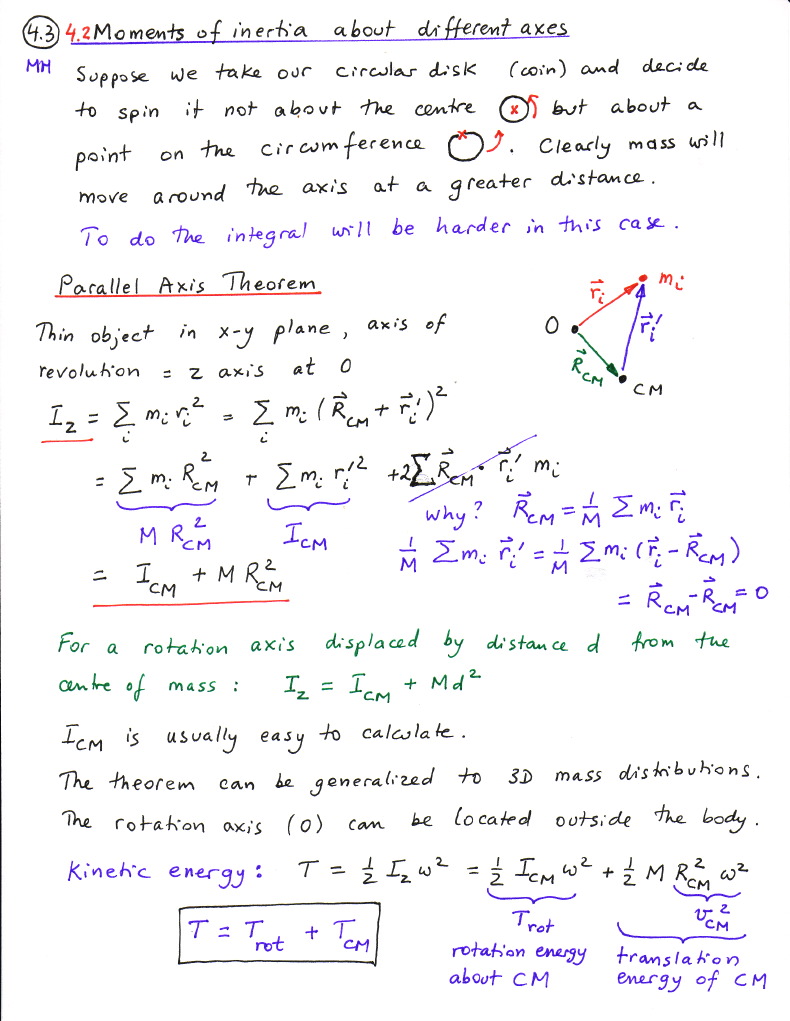
4.2 Moments of inertia about different axes
4.3 Torsion pendulum and physical pendulum
4.4 Arbitrary rigid body in rotation around fixed axis
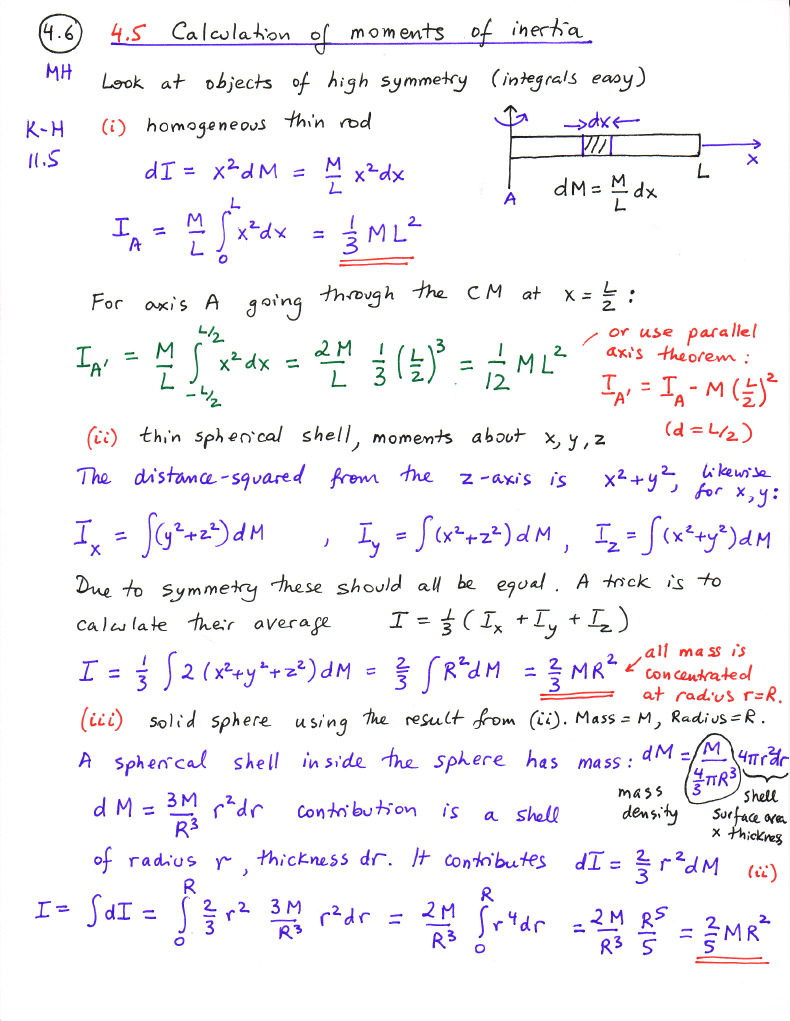
4.5 Calculation of moments of inertia
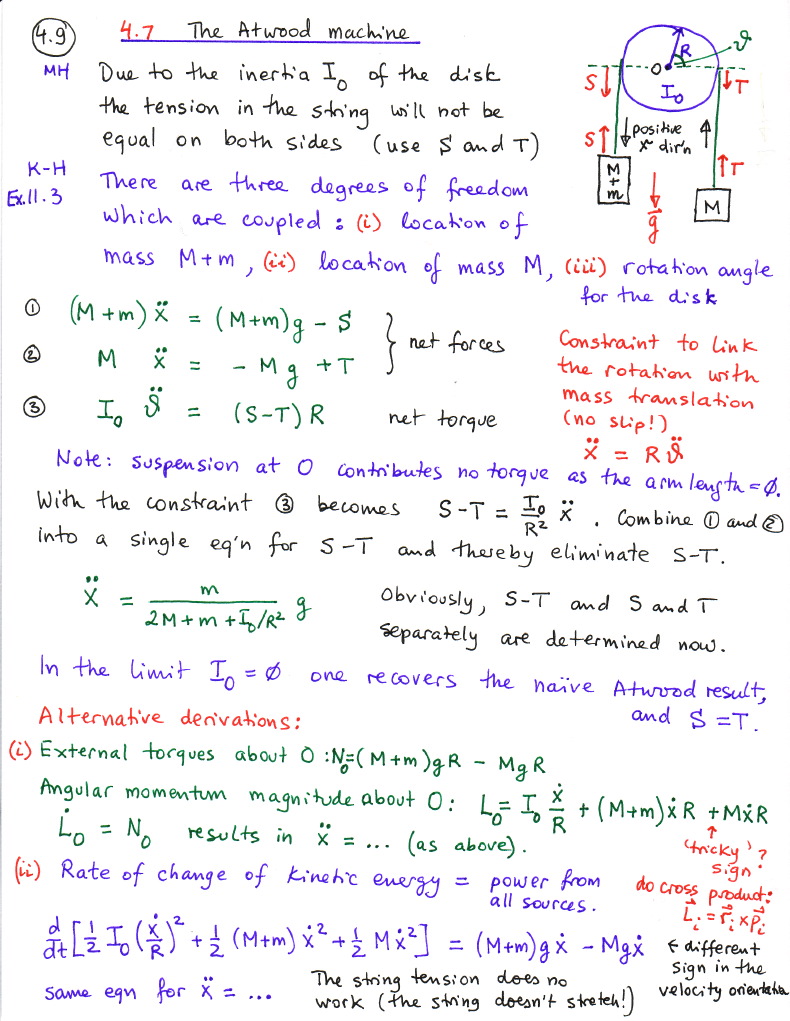
4.6 Equation of motion for rotation about fixed axis Rotational energy consideration
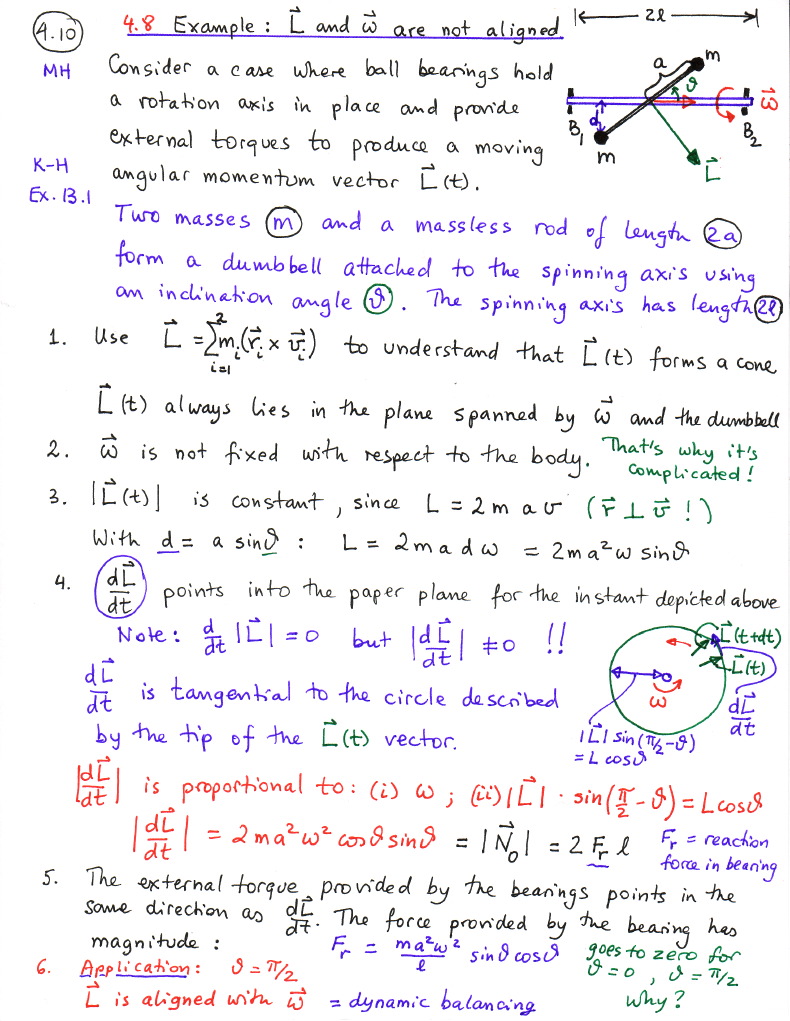
4.8 Example: when L and w are not aligned
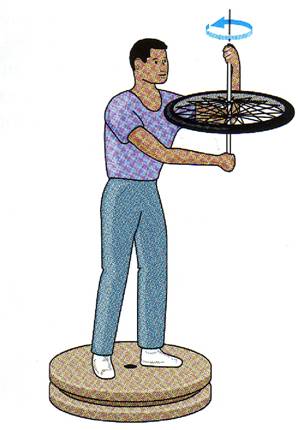
4.9 Example: precession in the gyroscope
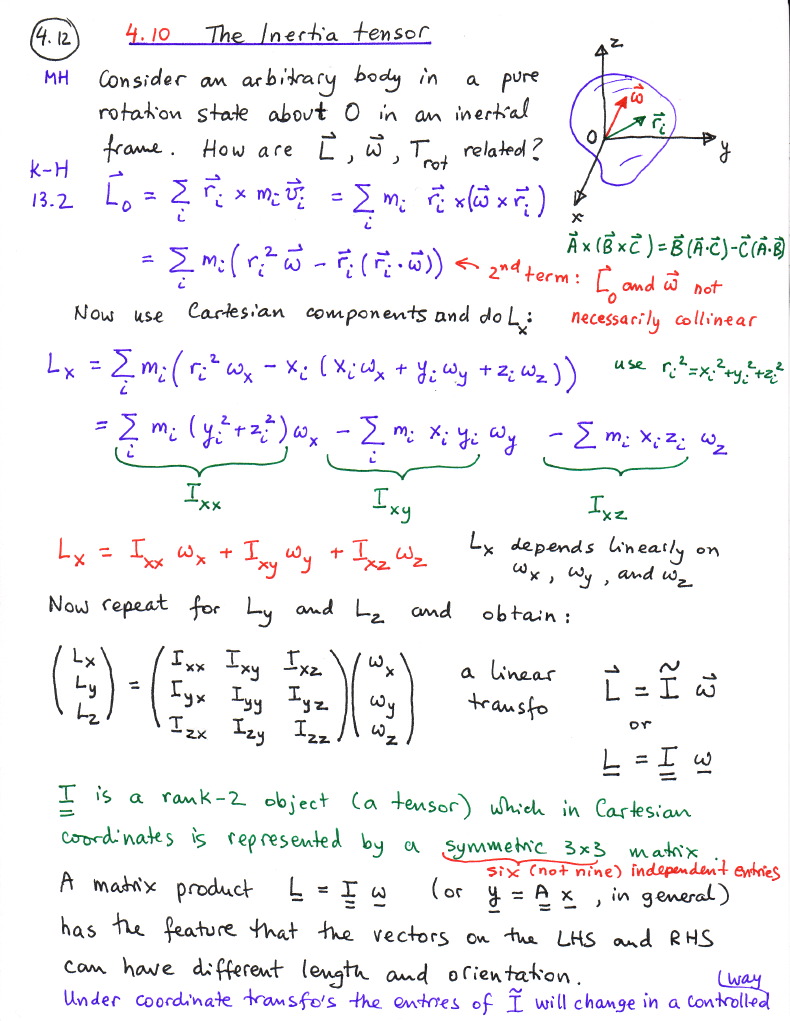
4.10 The Inertia tensor
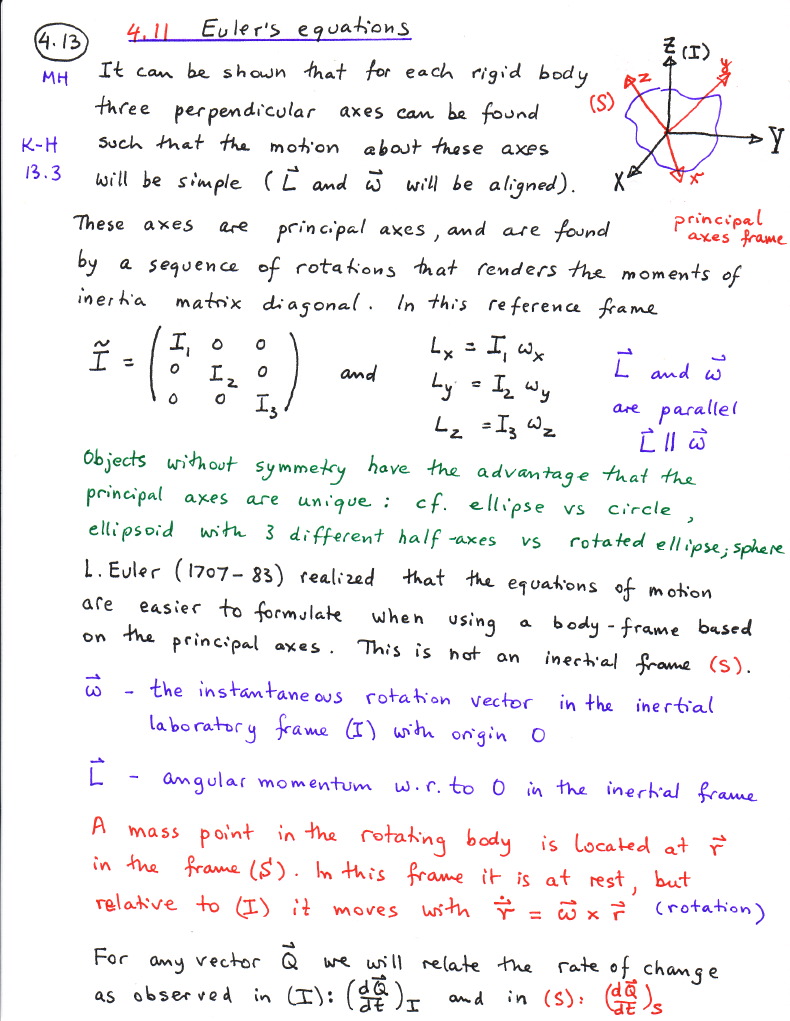
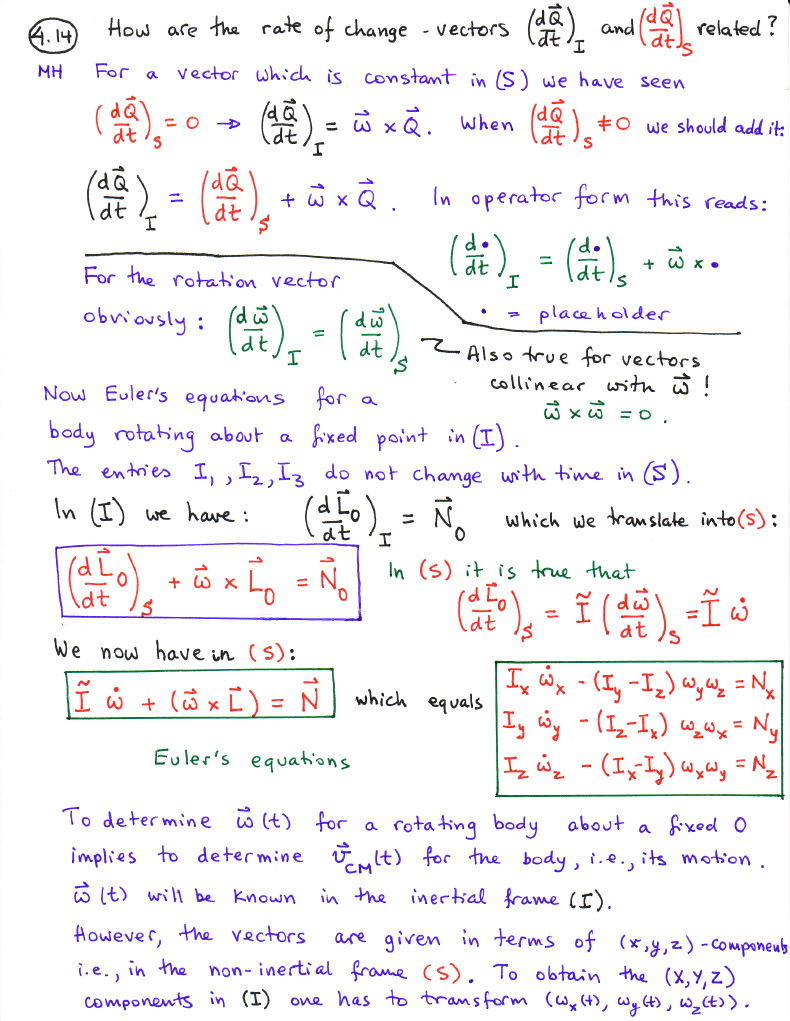
4.11 Euler’s equations Derivation
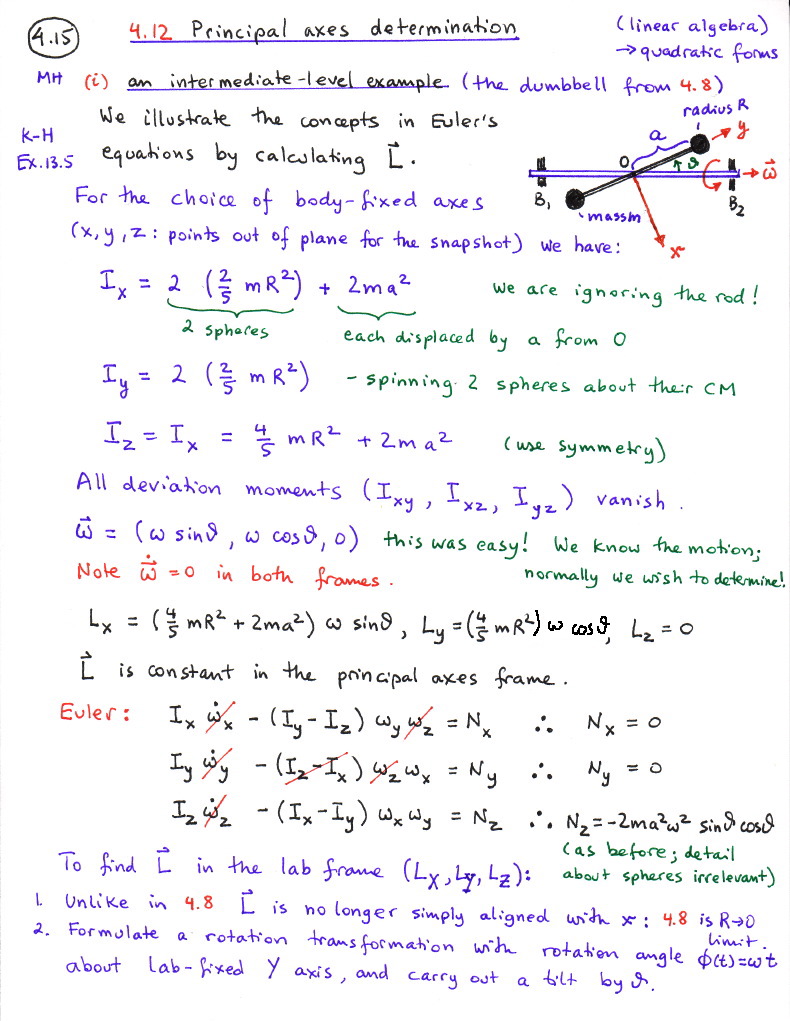
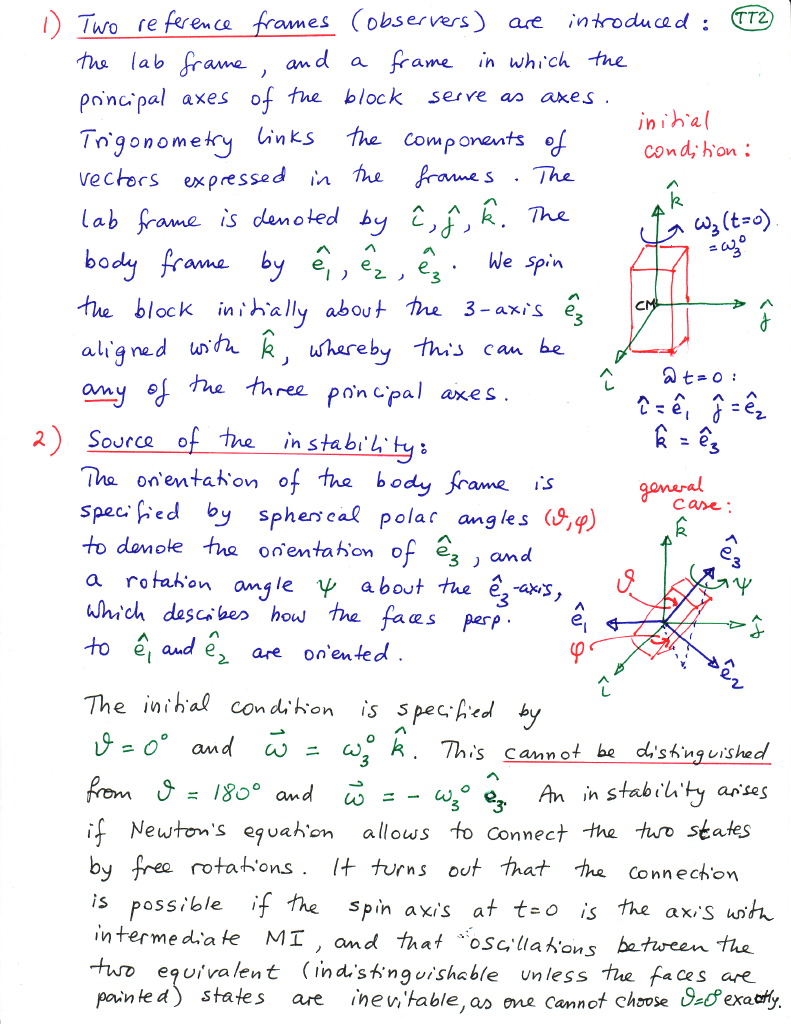
4.12 Principal axes determination example General case
Tumbling Textbook using Energy and Angular Momentum
Conservation
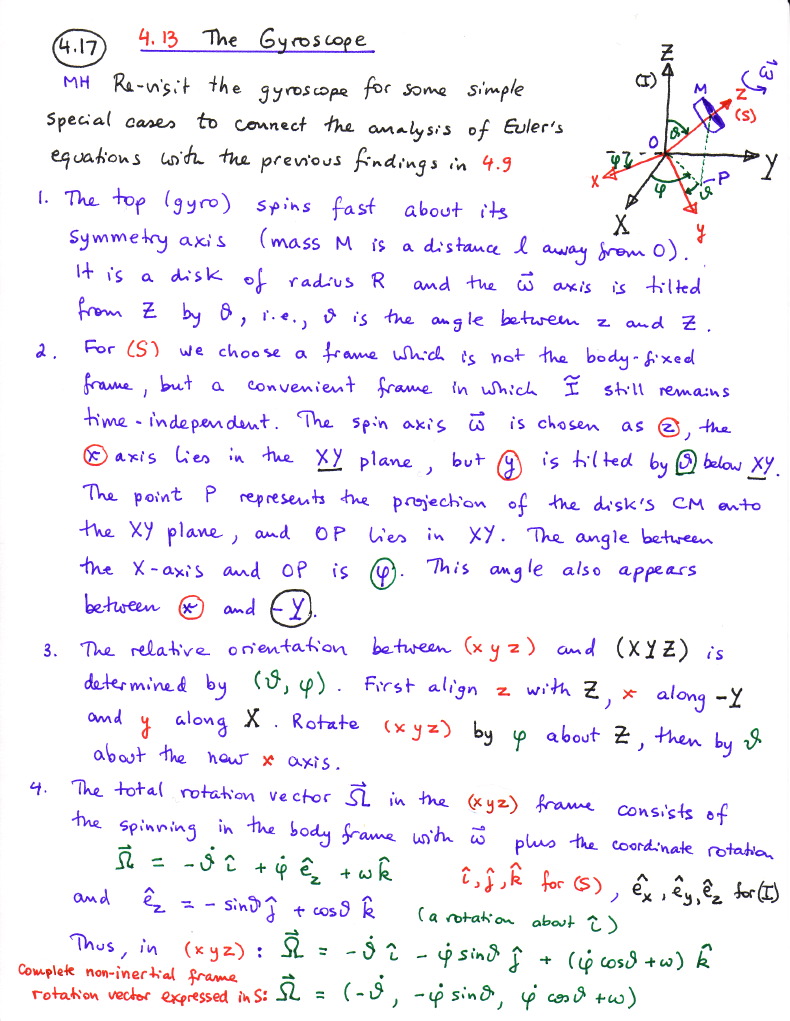
4.13 Gyroscope Simple cases
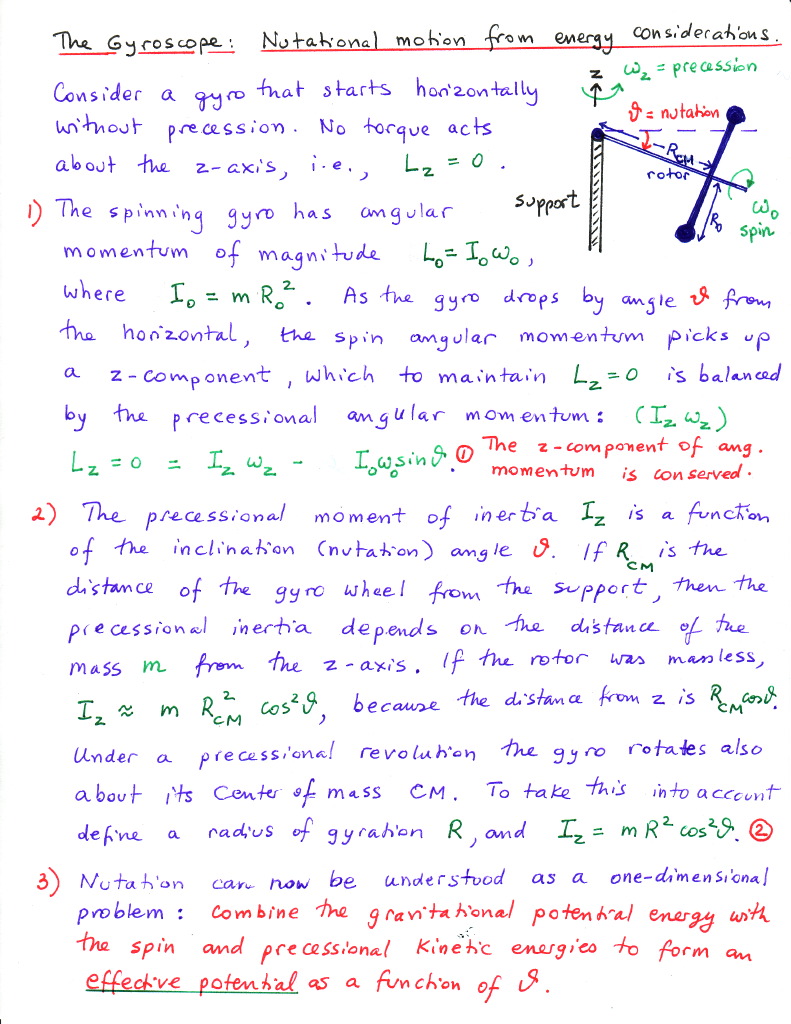
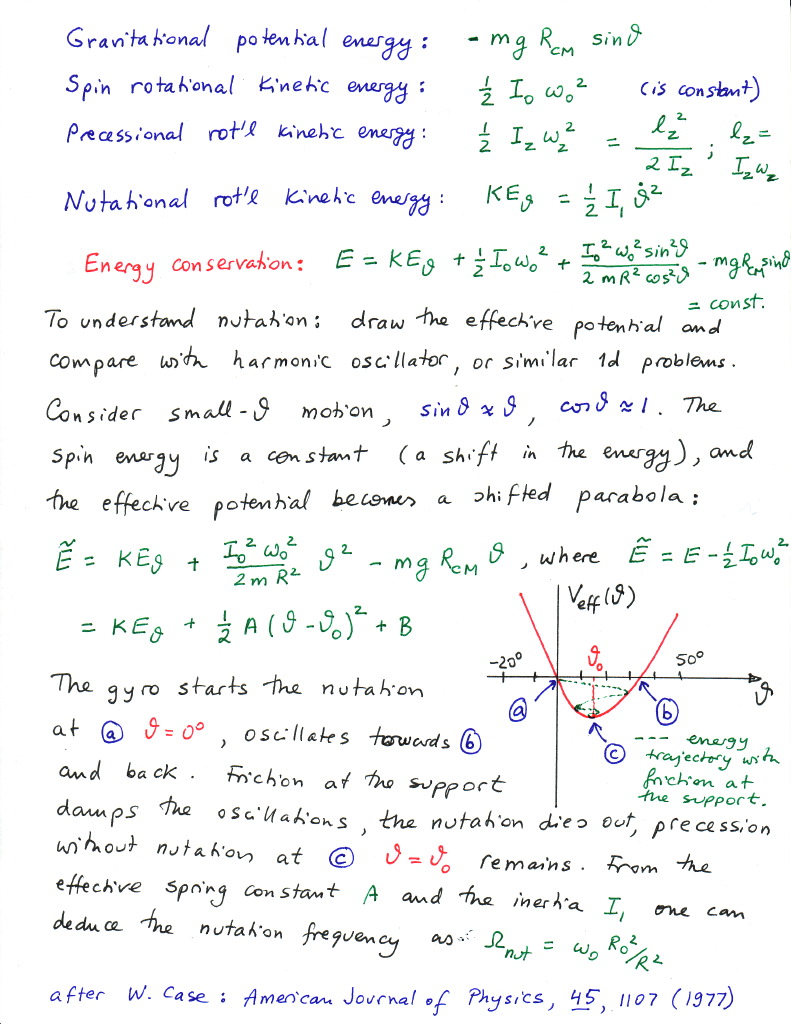
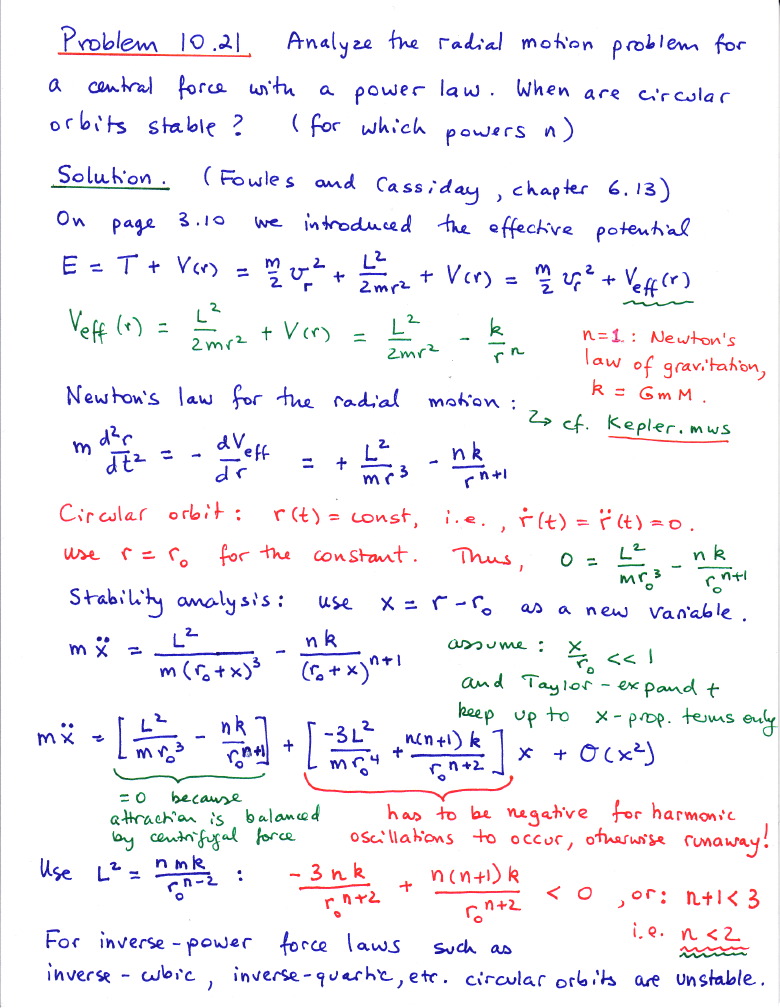
Nutation from Energy Considerations
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|

|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|